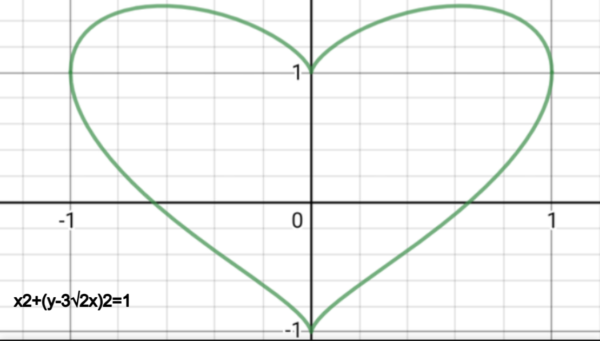
In math, there are some tricky problems called equations that we need to solve. One of these equations is x2+(y-3√2x)2=1 meaning (The equation represents a special shape called an ellipse, but it may look different from what you are used to seeing). It may seem hard at first, but if we understand it, we can learn some cool things and use it in real-life situations.
The equation x squared plus (y minus three times the square root of two times x) squared equals one means that if we plug in different values for x and y into the equation, we can find points on a special shape called an ellipse.
Also Read: Cos 180 Degree
What is the x2+(y-3√2x)2=1 Meaning and basics rule?
Before we learn more about the equation and how it is used, let’s carefully break it down and understand each part so we can have a good understanding.
Way the circle equation looks is like a standard form.
This equation is like a special code that tells us about a circle. The code says that the circle is centered at a point called the origin, which is like the very middle of a graph. The circle has a special size called a radius, and in this case, the radius is 1 unit long.
Breaking Down the x2+(y-3√2x)2=1 Equation into its Constituent Parts
Breaking down the equation means taking it apart and looking at each piece separately. It’s like taking a puzzle apart to see how each piece fits together.
To understand the equation, let’s break it down and look at each part separately.
- x^2: This component symbolizes the square of the x-coordinate of any point on the circle.
- (y – 3√2x)^2: Within this component, find the square of the difference of three times the square root of 2 multiplied by the y coordinate and the x coordinate of a point on the circle.
- = 1: This element means that the sum of the squares of these two components must be exactly equal to the number 1. This is essentially the square of the radius of the circle.
Graphic representation: a visual journey
To help you understand this x2+(y-3√2x)2=1 equation better, let’s draw it on a graph solution.

Visualizing the Circular Geometry means being able to imagine and understand the shapes and patterns that come from circles. It’s like being able to see and picture how circles can be used to create different things, like a wheel or a pizza.
When we draw this equation on a graph, we see a beautiful circle with a radius of 1 unit. The circle is perfectly centered at the point where the x and y axes meet. Basically, the equation helps us find all the points on the circle that fit its rules.
The geometric center is like the middle point of a shape, and the radii are like lines that go from the center to the edge of the shape.
In above picture of a circle, the center is the most important part and stays in one spot at the middle. The circle expands outwards from the center to a distance of 1 unit. Any point on the edge of the circle follows a specific math rule.
Practical Applications: Using math in everyday life.
Now that we have figured out what this equation means and how to draw it, we can start exploring all the cool things it can be used for in different areas.
Electrical engineering is like solving puzzles with electricity. Engineers use their knowledge to create and design things that use electricity, like computers and phones. They have to be very careful and precise in their work to make sure everything works correctly. It’s like drawing inside the lines to make sure everything looks nice and works well.
x2+(y-3√2x)2=1 equation Help in Electrical Engineering: Precision Boundaries
This equation is really helpful for electrical engineers. They use it to figure out exactly where things should go on circuit boards. It’s like drawing lines to show where everything should be placed.
x2+(y-3√2x)2=1 equation Help in Physics: The Trajectory of Particles
Physics is a type of science that helps us understand how things move and interact. One thing that physics helps us understand is the path that tiny particles take when they are thrown or shot through the air. We can figure out where these particles will go by studying their movement and using some special math.
In the world of physics, there is an equation that shows the path of a moving object in a circle. The equation looks like x squared plus (y minus 3 times the square root of 2 times x) squared equals 1. This equation tells us where the object is at any moment while it moves in a circle. It helps us understand how the object moves in its circular path.
In this activity, we will be making round mirrors and lenses using shapes and measurements.
x2+(y-3√2x)2=1 equation role in Geometry: Crafting Circular Mirrors and Lenses
The equation helps us make round shapes in geometry, which is useful for making things like round mirrors and lenses. These things are important for making instruments that help us see better. The equation is really important for making sure these instruments are made correctly.
Conclusion
The conclusion is like the ending of a story that explains the important meaning of the equation.
In simpler words, the equation x2+(y-3√2x)2=1 is actually a way to describe a circle. This equation is important and can be used in many different subjects like electricity, physics, and shapes. It helps us understand and solve problems in these areas.
