The a3+b3 formula, also known as the sum of cubes formula, is a math formula that helps us find the sum of the cubes of two numbers without having to actually calculate their cubes. It is also used to find the factors of a3 + b3, which can make math problems easier to solve.
In this article, we will learn about a formula called a3−b3. It goes like this: (a−b)(a2 + b2 + ab). There is also another formula called a3+b3 formula, which is a3+b3 = 3ab(a + b) – (a + b)3 or a3 + b3 = (a2 – ab + b2)(a + b). We will also look at some examples and more details about these formulas.
Also Read: 58: 2x^2 – 9x^2; 5 – 3x + Y + 6
What is a3 + b3 formula?
The a3 + b3 formula is a way to add or combine two numbers called a and b, by cubing each of them (multiplying them by themselves twice) and then adding the results together.
The a3 + b3 formula is a special way to find the total of two cubes without having to do the actual multiplication.
Let’s say we want to add 123 and 83, but we don’t want to actually add them together. We can use a special formula called a3+b3 formula to solve this without calculating the cubes of 8 and 12. This formula is helpful in solving math problems and some other types of problems too. You can see the formula in the picture provided.
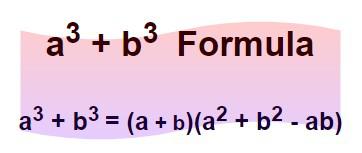
Adding the cube of a number a to the cube of another number b.
a3 + b3 Definition
The equation a3+b3 helps us find a way to break down or find the total of two numbers that have been cubed. We use this equation instead of actually calculating the cubes of the numbers. It comes from a longer formula called (a + b)3.
(a + b)3 = a3 + b3 + 3ab(a + b)
⇒ a3 + b3 = (a + b)3 – 3ab(a + b)
⇒ a3 + b3 = (a + b){(a + b)2 – 3ab}
⇒ a3 + b3 = (a + b){a2 + b2 + 2ab – 3ab}
⇒ a3 + b3 = (a + b)(a2 + b2 – ab)
When you add two numbers together and then multiply that sum by 3, it is the same as multiplying each of the original numbers by 3 and then adding them together, plus multiplying the two original numbers together and then multiplying that by 3.
When you add two numbers cubed, it’s the same as adding the two numbers together and cubing that sum, then subtracting 3 times the product of the two numbers and the sum of the two numbers.
When you add the cubes of two numbers, it’s equal to the sum of those two numbers multiplied by the square of their sum minus three times their product.
When you add the cubes of two numbers, it’s like multiplying their sum by the sum of their squares, with a couple of extra steps involved.
If you add the cube of number a to the cube of number b, it is the same as multiplying (a + b) by (a squared + b squared – a times b).
Factor of a3 + b3
A factor is a number that can be multiplied by another number to get a certain result. In this case, we are looking for numbers that can be multiplied together to get the expression a3 + b3.
To find the factors of a3 + b3, we use a special formula: (a + b)(a2 + b2 – ab). This formula helps us figure out what numbers or expressions we can multiply together to get a3 + b3. In simpler terms, factors are things that when multiplied together, give us the original number or expression. So in this case, the factors of a3 + b3 are…
Factors of a3 + b3 = (a + b)(a2 – ab + b2)
To find the factors of a number like a3 + b3, we can break it down into smaller parts. We can do this by multiplying two smaller numbers together. In this case, the factors are (a + b) and (a2 – ab + b2).
What is Sum of Cubes Formula?
The sum of cubes formula is a math equation that helps us find the total of three numbers that are all raised to the power of three.
The Sum of Cubes Formula helps us find the total value when we add two cubes together. It is also used to break down or simplify the expression of two cubes added together. The formula looks like this: a cubed plus b cubed.
When you add the cubes of two numbers, it’s the same as multiplying the sum of the two numbers with a special pattern.
a3 + b3 = (a + b)(a2 – ab + b2)
where,
a is the first variable
b is the second variable
This formula helps us find the total when we add together the cubes of two numbers, without having to figure out what the cubes are first.
a3 + b3 Formula Proof
The a3 + b3 formula is a special rule that helps us solve math problems. It says that if we have two numbers, a and b, and we want to find the answer when we raise them to the power of 3 (which means multiplying them by themselves three times), we can use this formula. This formula helps us find the sum of a cubed (a multiplied by itself three times) and b cubed (b multiplied by itself three times).
a3 + b3 = (a + b)(a2 – ab + b2)
RHS = (a + b)(a2 – ab + b2)
Using the Distributive Property of Multiplication, we have
RHS = a(a2 – ab + b2)) + b(a2 – ab + b2)
⇒ RHS = a3 – a2b + ab2 + a2b – ab2 + b3
⇒ RHS = a3 – a2b + a2b + ab2 – ab2 + b3
⇒ RHS = a3 – 0 + 0 + b3
⇒ RHS = a3 + b3
⇒ RHS = LHS
Hence proved.
(a + b)3 Formula
(a + b)3 formula is the formula that is used to expand the cube of sum of two numbers and the formula for the same is,
(a + b)3 = a3 + b3 + 3ab(a + b)
The sum of cube formula or a3 + b3 formula is a way to find the total when you add the cubes of two numbers together. To prove this formula, we solve the right-hand side (RHS) of the formula and simplify it until it is the same as the left-hand side (LHS).
When you add the cubes of two numbers, it’s the same as multiplying the sum of those numbers by the square of the first number minus the product of the two numbers plus the square of the second number.
The right-hand side equals the sum of two numbers multiplied by the sum of the square of the first number, minus the product of the two numbers, and the square of the second number.
The Distributive Property of Multiplication means that you can break up a multiplication problem into smaller parts and then add them together. For example, instead of multiplying 3 by 4 directly, you can break it up into 3 times 2 plus 3 times 2. Then you can add those two parts together to get the answer, which is 6 plus 6, which equals 12.
The right-hand side of the equation is equal to a bunch of numbers added together. The numbers are a cubed, minus a squared times b, plus a times b squared, plus a squared times b, minus a times b squared, plus b cubed.
The right-hand side of the equation is equal to a bunch of numbers and letters added together. It looks complicated, but if we simplify it, we can see that some parts cancel each other out.
On the right side, there are the cubes of two numbers, a and b.
On the right side, we have a cube made up of a and another cube made up of b.
The right side of the equation is equal to the left side of the equation.
That’s why we know it’s true.
To find the answer when you add a and b and then multiply it by 3, you can use this formula: (a + b) x 3.
The (a + b)3 formula is a way to find what happens when you multiply and add two numbers together three times. It helps us figure out what the answer will be.
When you add together two numbers, then multiply the result by 3, it’s the same as adding the cube of each number separately and then adding 3 times the product of the two numbers.
Adding the cubes of two numbers (a and b) together is different from subtracting the cubes of two numbers (a and b).
Both formulas a3 + b3 and a3 – b3 involve cubes, but they work in different ways. The table below shows the main differences between the two formulas.
Sum Best Examples on (a + b)3 Formula
Example 1: Factorize 343a3 + 216 using sum of cubes.
Solution:
Given,
343a3 + 216 = (7a)3 + (6)3
Since, a3 + b3 = (a + b)(a2 – ab + b2)
⇒ 343a3 + 216 = (7a + 6)[(7a)2 – (7a)(6) + (6)2]
⇒ 343a3 + 216 = (7a + 6)[49a2 – 42a + 36]
Example 2: Factorize 8p3 + 27 using sum of cubes.
Solution:
Given,
8p3 + 27 = (2p)3 + (3)3
Since, a3 + b3 = (a + b)(a2 – ab + b2)
⇒ 8p3 + 27 = (2p + 3)[(2p)2 – (2p)(3) + (3)2]
⇒ 8p3 + 27 = (2p + 3)[4p2 – 6p + 9]
